 The benefit of using a z-score in usability metrics was explained in “What’s a Z-Score and why use it in Usability Testing?” this article discusses different ways of calculating a z-score.
The benefit of using a z-score in usability metrics was explained in “What’s a Z-Score and why use it in Usability Testing?” this article discusses different ways of calculating a z-score.
The short answer is: It depends on your data and what you’re looking for. If you’ve encountered the z-score in a statistics book you usually get some formula like:

Calculating a Z-Score Example
For example, lets say you took the GRE a few weeks ago and got scores of 630 Verbal and 700 Quantitative. How good are these scores? Which is better, the Verbal or Quantitative score? Using a z-score can tell you how far you are from the mean and thus how well you performed. If you know the mean and standard deviations for a set of GRE test takers you can compare your scores.
ETS publishes the means and standard deviations of a set of test takers on the GRE website.
| Verbal | Quantitative | |
| Mean | 469 | 591 |
| StDev | 119 | 148 |
By plugging in your scores you get the following:
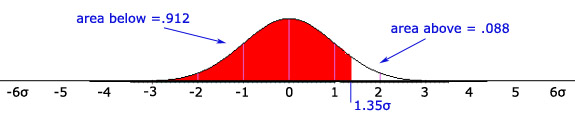
Verbal z = (630 – 469) ÷ 119 = 1.35σ
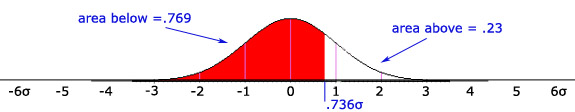
Quantitative z = (700 – 591) ÷ 148 = .736σ
To convert these sigma values into a percentage you can look them up in a standard z-table, use the Excel formula =NORMSDIST(1.35) or use the Z-Score to Percentile Calculator (choose 1-sided) and get the percentages : 91% Verbal and 77% Quantitative. You can see where your score falls within the sample of other test takers and also see that the verbal score was better than the quantitative score. Assuming the sample data was normally distributed, here’s how the scores would look graphically:
Figure 1: Verbal Score
Z-Scores and Process Sigma
An interactive Graph of the Standard Normal Curve similar to Figures 1 & 2 is available for you to visualize how the z-scores and the area under the normal curve correspond. The graphs also allow you to see the difference between one and two-sided (also called two-tailed) areas. In Six Sigma the process sigma metric is derived using the same method as a z-score. However, in Six Sigma you are measuring the distance a sample mean is above a specification limit–there can be an upper and lower spec limit that a sample must fall between as well. As in the z-score, you still use the same normal-deviates from the z-table to approximate the area under the curve. The process sigma metric is essentially a Z equivalent.
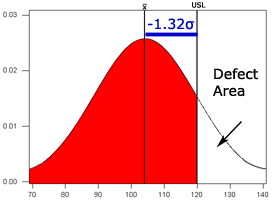
When testing software with users, task times are usually a good metric that will reveal the individual differences in performance. For task times there typically is only an upper spec limit. That is, it usually doesn’t matter how fast a user completes a task, but it does matter if a user takes too long. For example, say you and your product team determined that a task should be completed in 120 seconds. 120 seconds becomes your Upper Spec Limit (USL). You sampled 10 users and got these task times:
| Sample |
| 100 99 101 125 100 123 96 90 98 116 |
| USL: 120 Mean: 104 StDev: 12 |
To calculate the process sigma you subtract the mean (104) of the sample from the target (120) and divide by the sample standard deviation (12). For Sample 1 the process sigma is -1.32σ. The visual representation of the data can be seen below:
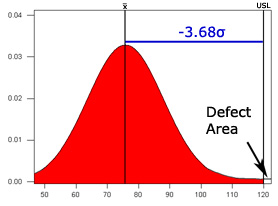
| Sample 2 |
| 60 75 99 88 65 72 75 72 87 65 |
| USL: 120 Mean: 75.8 StDev: 12.14 |
In the redesign, the average of the new sample is well below the spec limit and the process sigma is now very high. The corresponding defect area is now only .01% and the quality area is 99.98%
If you need more help with z-scores, see the Crash course in Z-scores, a tutorial with plenty of pictures, examples and review questions for you to grasp this concept.


